Answer:
The length of DD' is
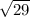 or 5.39 units.
or 5.39 units.
Explanation:
The distance formula is
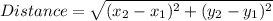
From the given figure it is clear that the coordinates of D are (2,0) and coordinates of D' are (7,2).
Using distance formula we get
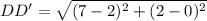
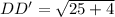

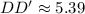
Therefore the length of DD' is
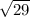 or 5.39 units.
or 5.39 units.