Question

By what percent will a fraction decrease if its numerator is decreased by 50% and its denominator is decreased by 25%?

AND NO, it is not 33, 33.3, 66, or 66.6 percent.

Answer 1

Let N be the numerator, D be the denominator

`(N)/(D)`

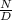
if its numerator is decreased by 50%

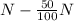
`N - (50)/(100)N` ==> (this will be the numerator part)

if its denominator is decreased by 25%

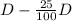
`D - (25)/(100)D` ==> (this will be the denominator part)

I try to separate them out, so it's easier to see the problem.


`N - (50)/(100)N = (100N)/(100) - (50N)/(100) = (50N)/(100)`


`D - (25)/(100)D = (100D)/(100) - (25D)/(100) = (75D)/(100)`


`((50N)/(100))/((75D)/(100)) = (50N)/(100) * (100)/(75D)`


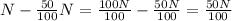
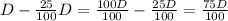
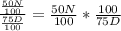
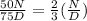
`(50N)/(75D) =  (2)/(3)((N)/(D))` by this, you can understand that

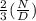
`(2)/(3)((N)/(D))` as 66.6% of N/D because 2/3 is 0.666666.... The answer should be 66.7% if you round them up.

Answer 2

The answer is 66.6666%