Outside the radius of the sphere, the electric field generated by a charged sphere (with charge Q on its surface) at a distance r from the centre is equivalent to the electric field generated by a single-point charge with total charge Q:
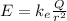
The problem asks to find the electric field at r=8.64 cm, while the radius of the sphere is R=5.00 cm, so r>R and we are exactly in this condition.
Re-arranging the previous formula, we can solve to find the total charge Q on the sphere. Using

and
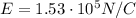
, we find
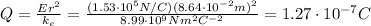
This is the total charge on the surface. If we want to find the number of electrons composing this charge, we should divide the total charge by the charge of a single electron, e:
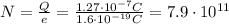
and this is the number of electrons.