Question

a baseball coach uses a pitching machine to simulate pop flies during practice. the quadratic function f(x)=-16x^2+70x+10 models the height in feet of a baseball after x seconds. how long is the baseball in the air if the ball is not caught?

Answer 1

Using the quadratic equation f(x) = -16
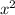`x^2` + 70x + 10, which models the height of the baseball, we apply the quadratic formula to find that the ball is in the air for approximately 4.438 seconds before it lands back on the ground if not caught.

Step-by-step explanation:

The student asks how long a baseball remains in the air during practice using a quadratic equation that models the height of the baseball after a certain number of seconds. The equation provided is f(x) = -16
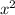`x^2` + 70x + 10, which models the height in feet of the baseball after x seconds. To determine the time the baseball is in the air, we need to find when the ball returns to the ground level (when f(x) = 0).

We can solve this quadratic equation using the quadratic formula: x = (-b ±
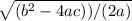`√((b^2 - 4ac)) / (2a))`, where a is -16, b is 70, and c is 10. Plugging in these values, we get two solutions for t (time), and we choose the positive time value at which the height becomes zero again, indicating that the ball has landed back on the ground.

The use of the quadratic formula yields t = 4.438 seconds and t = -0.138 seconds. We discard the negative time value as it is not physically relevant to the problem, and thus the baseball is in the air for approximately 4.438 seconds before it lands back on the ground if it's not caught.

Answer 2

We want to find where the function is 0. We will use the quadratic formula to solve this:

`x= (-b \pm √(b^2-4ac))/(2a)`
In this equation, a=-16, b=70 and c=10:

`x= (-70\pm √(70^2-4(-16)(10)))/(2(-16)) \\ \\= (-70 \pm √(4900--640))/(-32) \\ \\= (-70 \pm √(4900+640))/(-32) \\ \\= (-70 \pm √(5540))/(-32) \\ \\= (-70 \pm 74.43)/(-32) = (-70+74.43)/(-32) \text{ or } (-70-74.43)/(-32) \\ \\= (4.43)/(-32) \text{ or } (-144.43)/(-32) \\ \\=-0.138 \text{ or } 4.513`
Since a negative number makes no sense in the problem situation, we have a time of 4.513 seconds.