Answer:
see explanation
Explanation:
Using the product rule
Given
y = f(x)g(x) , then
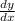 = f(x).g'(x) + g(x).f'(x) ← product rule
= f(x).g'(x) + g(x).f'(x) ← product rule
(a)
let
f(x) = (3x - 1)³ , then using the chain rule
f'(x) = 3(3x - 1)² ×
 (3x - 1) = 3(3x - 1)² × 3 = 9(3x - 1)²
(3x - 1) = 3(3x - 1)² × 3 = 9(3x - 1)²
let
g(x) = 2x + 5 ⇒ g'(x) = 2
Then
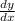 = (3x - 1)³ . 2 + (2x + 5) × 9(3x - 1)²
= (3x - 1)³ . 2 + (2x + 5) × 9(3x - 1)²
= 2(3x - 1)³ +9(3x - 1)²(2x + 5) ← factor out (3x - 1)²
= (3x - 1)²[ 2(3x - 1) + 9(2x + 5) ]
= (3x - 1)² [ 6x - 2 + 18x + 45 ]
= (3x - 1)²(24x + 43)
--------------------------------------------------------------
(b)
let
f(x) = 3x - 2 ⇒ f'(x) = 3
let
g(x) = 2x² + 3 ⇒ g'(x) = 4x
Then
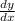 = (3x - 2).4x + (2x² + 3). 3 ← distribute parenthesis
= (3x - 2).4x + (2x² + 3). 3 ← distribute parenthesis
= 12x² - 8x + 6x² + 9
= 12x² - 8x + 9
Note
It is usual to simplify after differentiating the expressions