Question

How are solutions, roots, and x-intercepts of a quadratic related?

Answer 1

Explanation:

The quadratic equation
`ax^2+bx+c=0`

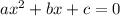
The roots of quadratic equation is defined as that value when we substitute in the quadratic equation then we get zero.

Solution of quadratic equation is defined as that value of roots which substitute in the equation then we get zero.

x-intercept of the quadratic equation is defined as that real value of x on the x- axis when we substitute in the equation then the value of equation becomes zero.

From the above definitions we can see that roots of quadratic equation can be imaginary or real but x- intercept value of equation is always a real value.

Solution is also called the value of roots but x- intercept is equal to roots when the value of roots is real.

Now, we can says that roots and solutions of quadratic equation are same when values of roots are real. But when the roots are imaginary then we can not find x- intercept in cartesian coordinate plane .

Answer 2

A quadratic equation has the form ax2+bx+c=0 and is represented by a parabola (graphically). The solution of a quadratic equation is based on find the roots, which are the x-intercepts of its graph. When it has double root, the vertex of the parabola is the intercept with the x axis. If the root is imaginary (the discriminant of a quadratic function is negative), it does not intercept the x axis. So, a quadratic equation can has: one root, two roots, or zero roots.

You can solve a quadratic equation by factoring by applying a equation called "Quadratic formula", which is usually used when you can't factorize it. This formula is:

x=(-b±√(b^2-4ac))/2a