Question

A woman falls to the ground while wearing a parachute. The air resistance on the parachute of the parachute is 500N. If the woman falls at a constant rate of 5m/s, then the gravitational force on her is

Answer 1

The correct answer is A.

Step-by-step explanation:

Answer 2

The fact that the woman falls at a constant velocity means its acceleration is zero.
For Newton's second law, the resultant of the forces acting on a body is equal to the product between the mass m of the body and its acceleration a:

`\sum F = ma`
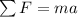
In our problem, the acceleration is zero, so the resultant of the forces should be zero as well.

Only two forces are acting on the woman: the air resistance R (upward, 500 N) and the weight W (downward). The resultant of these two forces is zero, so

`R-W=0`

`W=R`
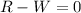
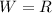
and since the air resistance R is 500 N, then the weight (the gravitational force) of the woman is 500 N as well.