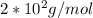Question

During an effusion experiment, oxygen gas passed through a tiny hole 2.5 times faster than the same number of moles of another gas under the same conditions. What is the molar mass of the unknown gas? (Note: the molar mass of oxygen gas is 32.0 g/mol.) 2.0 × 102 g

Answer 1

The molar mass of the unknown gas is 200 g/mol. R:
`2*10^2 g/mol`

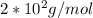
Step-by-step explanation:

We have an effusion experiment with oxygen and another unknown substance.

Oxygen effuses (v1) through a tiny hole 2.5 times faster than unknown substances (v2). It means

v1 = 2.5v2.

Molar mas of Oxygen is M1 = 32.0 g/mol.

This process can be studied by using Graham law.

`(v1)/(v2) = \sqrt[2]{(M2)/(M1) }`

Where M2 is the unknown molecular mass, all the other data are given in the problem. Replacing and isolating M2. we can fin its value:

`(v1)/(v2) = \sqrt[2]{(M2)/(M1) } \\v1 = 2.5 v2\\(2.5v2)/(v2) = \sqrt[2]{(M2)/(M1) } \\\\2.5 =  \sqrt[2]{(M2)/(M1) } \\(M2)/(M1) = (2.5)^2\\M2 = (2.5)^2 M1 = (2.5)^2*32.0 (g)/(mol)\\ M2 = 6.25* 32.0 (g)/(mol) = 200 (g)/(mol)\\M2 = 200 (g)/(mol)`

The molar mass of the unknown gas is 200 g/mol. R:
`2*10^2 g/mol`

Answer 2

Mass oxygen = 32.0 g/mol
Mass of unknown gas = ???

Rate of effusion unknown gas = x
Rate of effusion (O2) = 2.5 x

r1/r2 = sqrt(m2)/sqrt(m1)
2.5x/x = sqrt(unknown mass)/sqrt(oxygen mass)
2.5/1 = sqrt(unknown mass)/sqrt(32)
2.5 * sqrt(32) = sqrt(unknown) Square both sides.
6.25*32 = unknown mass

200 = mass of unknown gas.<<<=== answer