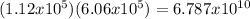Question

Select the problems which show the correct answers. (1.12 x 105) (6.06 x 105) Answer: 6.79 x 1010 (2 x 101)(3.0 x 101) Answer: 6.0 x 101 (2.775 x 10−4) (4.775 x 104) Answer: 1.325 x 100 5 8.14 x 102 Answer: 6.14 x 10−3

Answer 1

`(1.12 x 10^5) (6.06 x 10^5) = 6.787 x 10^(10)\\`

`(0.5 x 10 ^ 1)/(8.14 x 10 ^ 2) = 6.14 * 10 ^ {-3}\\`

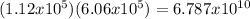
Explanation:

To solve this problem we must solve all 4 operations.

For the first one we have:

`(1.12 x 10^5) (6.06 x 10^5)`

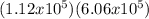
When we have multiplication of powers of equal base (10) the same base is placed and the exponents are added

So:

`1.12 * 6.06 x 10^(5+5) = 6.787 x 10^(10)\\`

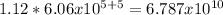
Therefore the answer to this question is correct.

For the second question we have:

`(2 x 10^1) (3.0 x 10^1)`

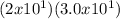
Then following the same procedure as in the previous question we have:

`2 * 3.0 x 10 ^ {1 + 1} = 6 x 10 ^ 2\\`

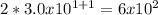
Then the answer shown in the statement is not correct, because 10 must be squared, product of the sum of its exponents (1 + 1)

For the third question we have:

`(2,775 x 10^(-4)) (4,775 x 10^4)\\2.775 * 4.775 x 10 ^((- 4) +4) = 13.251 x10 ^ 0\\`

Then it is verified that the answer for this operation is no correct

`13.25\\eq 1.325`

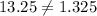
Finally, for the fourth question we have:

`(5)/((8.14 x 10 ^ 2))\\`

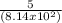
This we can write it as:

`(0.5 x 10 ^ 1)/(8.14 x 10 ^ 2)\\`

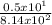
In division of powers of equal base (10 in this case) the same base is placed and the exponents are subtracted:

`\frac{0.5}{8.14 x 10 ^ {1-2}} = 0.0614 x 10 ^ {-1} = 6.14 * 10 ^ {-3}\\`

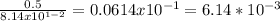
Finally, the problems that show correct answers are:

`(1.12 x 10^5) (6.06 x 10^5) = 6.787 x 10^(10)\\`

`(0.5 x 10 ^ 1)/(8.14 x 10 ^ 2) = 6.14 * 10 ^ {-3}\\`