Question

PLEASE SHOW ALL WORK!!!

The base of a triangle measures (8x + 2) units and the height measures (4x − 5) units.

Part A: What is the expression that represents the area of the triangle? Show your work to receive full credit. (4 points) Hint: Area = 0.5bh

Part B: What are the degree and classification of the expression obtained in Part A? (3 points)

Part C: How does Part A demonstrate the closure property for polynomials? (3 points)

Answer 1

Part A:

As given,

Base of the triangle = 8x + 2 units

Height of the triangle = 4x − 5 units

Formula to find area of a triangle =
`(base * height)/(2)`

= 0.5bh

we have been given base and height, so the area becomes,

area =
`((8x+2)(4x-5))/(2)`

area = 0.5(8x+2)(4x-5)

= 0.5(32x²-32x-10)

= 16x²-16-5

after factoring it, we get

area = (4x-5)(4x+1) units.

Part B:

It is a degree 2 polynomial as the polynomial has one variable that is x, and the largest exponent of that variable is 2.

Part C:

Part A demonstrates the closure property of polynomials because after multiplying two polynomials we get another polynomial.

Answer 2

Part A: We know that the equation for finding the area of a triangle is
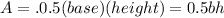
`A=.0.5(base)(height)=0.5bh`; we also know that
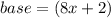
`base=(8x+2)` and
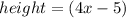
`height=(4x-5)`, so the only thing we need to do is replacing those values into our Area equation and solve for x:

`A=0.5(8x+2)(4x-5)`

`A=0.5(32 x^(2) -40x+8x-10)`

`A=0.5(32 x^(2) -32x-10)`

`A=16 x^(2) -16x-5`
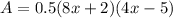
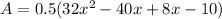
Now the only thing left is factor the quadratic polynomial:

`A=(4x+1)(4x-5)`

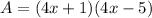
W can conclude that the area of our triangle is
`A=(4x+1)(4x-5)`

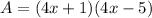Part B: Our polynomial has three terms, so is a trinomial; also, our polynoal only has one variable,
`x`, and the largest exponent of that variable is 2; therefore is a degree 2 polynomial. In summary, we have a trinomial of degree 2.

Part C: Part A demonstrate the closure property of polynomials because after multiplying tow polynomials we obtained another polynomial.