Answer:
![\displaystyle (d)/(dx)[3x + 5x] = 8](https://img.qammunity.org/2022/formulas/mathematics/college/zsxhhw1uujsjg1dfp5nxb0uweracmjiqgf.png)
General Formulas and Concepts:
Calculus
Differentiation
- Derivatives
- Derivative Notation
Derivative Property [Multiplied Constant]:
![\displaystyle (d)/(dx) [cf(x)] = c \cdot f'(x)](https://img.qammunity.org/2022/formulas/mathematics/high-school/rwpyhrof52dro5d128gleq5obchnuu5qkj.png)
Basic Power Rule:
- f(x) = cxⁿ
- f’(x) = c·nxⁿ⁻¹
Explanation:
Step 1: Define
Identify
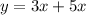
Step 2: Differentiate
- Simplify:
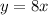
- Derivative Property [Multiplied Constant]:
![\displaystyle y' = 8(d)/(dx)[x]](https://img.qammunity.org/2022/formulas/mathematics/college/gaxry98akjitzdqaej878sc3abv8n9bnlo.png)
- Basic Power Rule:
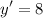
Topic: AP Calculus AB/BC (Calculus I/I + II)
Unit: Differentiation