Question

Which of the following is a polynomial function in factored form with zeros at 0, –3, and 4?

A. f(x) = x^3 + x^2 – 12x
B.

f(x) = x(x – 3)(x + 4)
C. f(x) = x^3 – x^2 – 12x
D. f(x) = x(x + 3)(x – 4)

Answer 1

The answer is D.

Step-by-step explanation:
You can find the zeroes of a polynomial function in factored form by setting each factor equal to zero and solve for
`x`.

The polynomial function of answer A is not even factored, so we can rule that one out.

Lets set each one of the factors of answer B equal to zero, solve for
`x` and see what happens:
-
`x=0`
-
`x-3=0`

`x=3`
-
`x+4=0`

`x=-4`
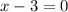
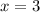
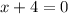
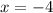
As you can see, our zeroes are 0,3, and -4, so this is not the correct answer either.

The polynomial function of answer C is not even factored, so we can rule that one out as well.

Lets apply what we just learned to the factored polynomial of answer D:
-
`x=0`
-
`x+3=0`

`x=-3`
-
`x-4=0`

`x=4`
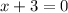
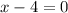
This time our zeroes are, 0, -3, and 4; therefore we can conclude that D is the correct answer.