Question

​ Quadrilateral ABCD ​ is inscribed in this circle. What is the measure of ∠A ? Enter your answer in the box.

Answer 1

Subtract 43 from 180 as the sum of a triangle adds up to 180 degrees.

180 - 43 = 137

:)

Answer 2

Answer: The answer is 137°.

Step-by-step explanation: Given that the quadrilateral ABCD is inscribed in a circle, where measure of angle C is 43°. We are to find the measure of ∠A.

Since the quadrilateral ABCD is inscribed in a circle, so it will be a cyclic quadrilateral. Also, the sum of opposite angles of a cyclic quadrilateral is 180°.

Therefore, we have

`\angle A+\angle C=180^\circ\\\\\Rightarrow \angle A+43^\circ=180^\circ\\\\\Rightarrow \angle A=180^\circ-43^\circ\\\\\Rightarrow \angle A=137^\circ.`

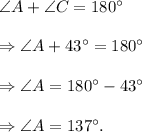
Thus, the required answer is 137°.