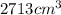Answer:
Explanation:
From the given figure, the radius of the cylinder is=8cm, height of cylinder is =18cm and the radius of the sphere is= 6cm.
Thus,
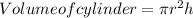
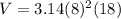
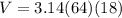

And
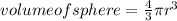
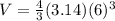
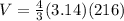

Thus, the volume of the shaded part of the figure=Volume of cylinder-volume of sphere
⇒volume of the shaded part of the figure=
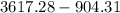
=
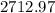
≈
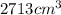
Thus,volume of the shaded part of the figure=