Question

In the diagram below, AC is perpendicular to BD. Find X.

Answer 1

6x - 15 = 90
6x = 90 + 15
6x = 105
x = 105/6
x = 17.5

Answer 2

Answer: The correct option is

(D) 17.5.

Step-by-step explanation: Given that in the diagram shown, AC is perpendicular to BD, where

`m\angle BEC=(6x-15)^\circ,\\\\m\angle AED=(3y+12)^\circ.`

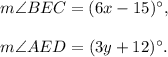
We are to find the value of x.

Since AC is perpendicular to BD and they are intersecting each other at the point E.

So, all the four angles made by this intersection are right angles.

That is, angle BEC is also a right angle.

Therefore, we get

`m\angle BEC=90^circ\\\\\Rightarrow (6x-15)^\circ=90^\circ\\\\\Rightarrow 6x-15=90\\\\\Rightarrow 6x=105\\\\\Rightarrow x=(105)/(6)\\\\\Rightarrow x=17.5.`

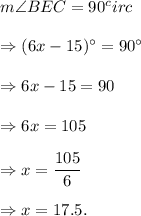
Thus, the required value of x is 17.5.

Option (D) is CORRECT.