Answer: The length of the hypotenuse is 5√34 units.
Explanation: Given that a right-angled triangle has legs of length 15 ft and 25 ft.
We are to find the length of the hypotenuse.
As shown in the attached figure, AB and BC are the legs of the right-angled triangle ABC. AC is the hypotenuse of the triangle.
Also, AB = 15 ft and BC = 25 ft.
From Pythagoras theorem, we have
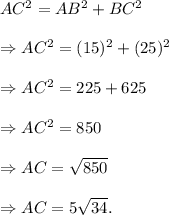
Thus, the length of the hypotenuse is 5√34 units.