Question

What are the domain and range of the function f(x)=1/4(x^3+6x^2+5x-4)

Answer 1

d all infinities

Explanation:

Answer 2

Domain and Range of f(x) is all real numbers i.e
`(-\infty, \infty)`

Option 4 is correct.

Explanation:

Given the function

`f(x)=(1)/(4)(x^3+6x^2+5x-4)`

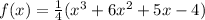
we have to find domain and range of the above function.

The domain of a function is the complete set of possible values of the independent variable i.e of x. The denominator function can't be equals to 0 when taking the values of x.

`f(x)=(1)/(4)(x^3+6x^2+5x-4)`

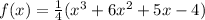
Here the function defines at each and every value of x therefore

Domain of f(x) is all real numbers i.e
`(-\infty, \infty)`

The range of a function is the complete set of all resulting values of the dependent variable i.e of y, after substituting the values of the domain.

Range of f(x) is all real numbers i.e
`(-\infty, \infty)`

Option 4 is correct.