Question

Analyze the key features of the graph of the quadratic function f(x) = -x2 + 2x + 10. A. Does the parabola open up or down?

B. Is the vertex a minimum or a maximum?
C. Identify the axis of symmetry, vertex and the y-intercept of the parabola.

Answer 1

SOS

Answer

A: Down

B: Max

C:

1. AOS: x = 1
2. Vertex: (1, 11)
3. Y-Int: (0, 10)

Explanation:

A: If the leading coefficient (a) is positive, the graph opens UP. If it is negative, the graph opens DOWN.

B: Anytime the graph goes UP, there's a minimum. Anytime the graph goes DOWN, there's a maximum

C:

AOS:
`x = (-b)/(2a), a=-1, b=2, c=10`

Vertex:
`((-b)/(2a), y)`

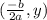
Y-INT: let x=0 and solve for y. The coordinate of the y-intercept is (0, y).

Hope this helps!!

Answer 2

see the attached graphic

the parabola open down
the vertex is a maximum
the axis of symmetry is the y axis
the y-intercept of the parabola.is the point (0,10)