Answer:
The y-intercept is (0,210)
Explanation:
We have the function,

So, we know that,
'y-intercept is the point on the graph where the function crosses y-axis'.
i.e. y-intercept is obtained when x=0.
Thus, substituting x=0 in the given function, we get,
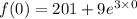
i.e.
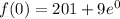
i.e.
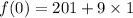
i.e.
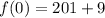
i.e. f(0) = 210
Also, we can see from the given graph that the function crosses y-axis at (0,210).
Hence, the y-intercept is (0,210).