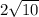On examining the sides of the parallelogram, we see that the side KL lies in the plane x=1, and the side MN lies in the plane x=3.
Hence the height of the parallelogram is h=(3-1)=2.
The length of side mKL=sqrt((5-2)^2+(3-2)^2)=sqrt(3^2+1^2)=sqrt(10)
The length of side mMN=sqrt((11-8)^2+(3-2)^2)=sqrt(3^2+1^2)=sqrt(10)
Therefore the area of the parallelogram is mKL*h = sqrt(10)*2 = 2sqrt(10)
Answer: Area of parallelogram =