In order to solve this quadratic equation by completing the square, let's analyse the coefficient b by comparing the equation with the standard form:
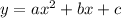
We have b = -12. In order to be a perfect square, the coefficient c needs to be (-12/2)^2 = (-6)^2 = 36
We already have 30, so we just need to add 6:
![\begin{gathered} x^2-12x+30=0 \\ x^2-12x+30+6-6=0 \\ x^2-12x+36-6=0 \\ (x-6)^2-6=0 \\ (x-6)^2=6 \\ x-6=\pm\sqrt[]{6} \\ x_1=6+\sqrt[]{6} \\ x_2=6-\sqrt[]{6} \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/high-school/jl8jufb0gipt6cu486rg.png)