Question

Pearl deposited $60 into a savings account for which interest is compounded monthly. According to the rule of 72, what interest rate will cause her money to double in approximately 33 years? A. 2.2% B. 0.6% C. 0.5% D. 1.8%

Answer 1

The rule of 72 says that the time it takes to double an investment is approximately equal to 72 divided by the interest rate in %.

Thus
33 years =72/i => i=72/33=2.18%, or approximately 2.2%.

Answer 2

A. 2.2% is correct.

Explanation:

Deposit Amount = $60

Pearl saving $60 into an account with interest is compounded monthly.

Her money is double in approximately 33 years.

Formula

`A=P\left ( 1+(r)/(n) \right )^(n* t)`

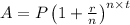
where,

A is final amount. (Double of initial value). A=$120

P is deposit amount. P=$60

r is rate of interest. r=?

n number of period. n=12

t is total time. t=33

Substitute all these values into formula.

`120=60\left ( 1+(r)/(1200) \right )^(12* 33)`

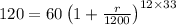
Now we solve for r

`\left ( 1+(r)/(1200) \right )^(396)=2`

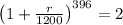
Taking log both sides

`396\log\left ( 1+(r)/(1200) \right )=\log 2`

`\log\left ( 1+(r)/(1200) \right )=0.00076`

`1+(r)/(1200)=10^(0.00076)`

`r=1200(1.00179-1)`

`r=2.2\%`

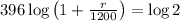
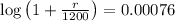
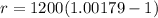
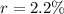
Thus, Rate of interest is 2.2%