Question

A 30 Ω resistor and a 60 Ω resistor are connected in parallel and placed across a potential difference of 120V. what is the equivalent resistance of the circuit? show work.

Answer 1

The resistance of a parallel network is given by


`R_(total)= (1)/( (1)/(30 \Omega)+ (1)/(60 \Omega) ) =20 \Omega`

Answer 2

Here we have to calculate the total equivalent resistance and the expression for work

As per the question the two resistors are connected as parallel in a circuit.

Let
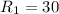`R_(1) = 30`ohm and
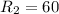`R_(2) =60`ohm

Let R is the total equivalent resistance

Hence
`(1)/(R) =(1)/(R_(1) ) +(1)/(R_(2) )`

⇒
`R=(R_(1)*R_(2)  )/(R_(1)+R_(2)  )`

=
`(30*60)/(30+60)`

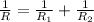
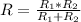
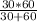
=20 ohm

Here the potential difference is given as V=120 volt

Hence the current flowing through the wire is given as

`I=(V)/(R)`

=
`(120 volt)/(20 ohm)`

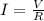
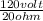
=6 ampere[6A]

The work done is equal to the electric energy consumed by the circuit.

Let t is the time of consumption .Hence the work done is given as

`W=I^(2)Rt`

=
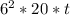`6^(2) *20*t` J

= 720t J

Taking various time of consumption we can get the work done