Question

Find x and y, given that line WS and line VT are parallel. Show all work!!

Answer 1

Given that those lines are parallel they will form similar traingles. This means the lengths of the sides of the triangles will be proportional through a ratio.

Find the ratio: divde the given small side by the given big side(they must be the same sides of the triangles though)

For example:
I found the ratio by dividing 8 / (20) and simplify to 2/5

Next step: multiply 22 by the fraction to get "y"

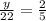
`(y)/(22) = (2)/(5)`
22*
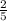
`(2)/(5)`= y
y=8.8 units


To find x :

Step 1: write an equation for the length of side US.
US= 5+x-2= x+3

Step 2: DIVIDE 5 by the ratio (must divide to get longer side DO NOT multiply)
US= 5/(2/5)= 12.5 units

Step 3: set the expression you found for US equal to 12.5

12.5= x+3
subtract 3 from both sides to isolate x.
x= 9.5 units

My answers:
x= 9.5 units
y= 8.8 units