Question

The cost function for a certain company is C = 20x + 700 and the revenue is given by R = 100x − 0.5x2. Recall that profit is revenue minus cost. Set up a quadratic equation and find two values of x (production level) that will create a profit of $700.

Answer 1

x = 20

x = 140

Explanation:

Find the equation for profit P

P = R - C

Substitute the equations for R and C then simplify.

P = 100x − 0.5x² - (20x + 700)

P = -0.5x² + 80x - 700

Find the values of x when profit is $700

P = -0.5x² + 80x - 700

700 = -0.5x² + 80x - 700

0 = -0.5x² + 80x - 1400

This is in the standard form 0 = ax² + bx + c

Use the quadratic formula to find values of x

`x = \frac{-b±\sqrt{b^(2)-4ac}  }{2a}`

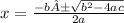
(Ignore Â)

Substitute a b and c.

a = -0.5

b = 80

c = -1400

`x = \frac{-(80)±\sqrt{80^(2)-4(-0.5)(-1400)}  }{2(-0.5)}`

`x = (-80±60)/(-1)`

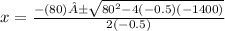
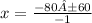
Split the formula at the ± so that there are two to get the two x values.

`x = (-80+60)/(-1)`

`x = (-80-60)/(-1)`

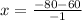
x = 20

x = 140

The profit will be $700 when x is 20 or when x is 140.