Question

Triangle ABC is similar to triangle PQR, as shown below:

Two similar triangles ABC and PQR are shown. Triangle ABC has sides AB equals c, BC equals a, and AC equals b. Triangle PQR has

Which equation is correct? (1 point)


c by a is equal to q by r

c by b is equal to a by r

b by q is equal to a by p

c by q is equal to a by r

Answer 1

Answer: The answer is (3) b by q is equal to a by p.

Step-by-step explanation: Given that ΔABC and ΔPQR are similar to each other.

Also, AB = c, BC = a, CA = b, PQ = r, QR = p and RP = q.

From the figure provided, we can conclude that the vertex A corresponds to vertex P, vertex B corresponds to vertex Q and vertex C corresponds to vertex R.

We know that the corresponding sides of two similar triangles are proportional.

Since ΔABC and ΔPQR are proportional, so we have

`(AB)/(PQ)=(BC)/(QR)=(CA)/(RP)\\\\\\\Rightarrow (c)/(r)=(a)/(p)=(b)/(q).`

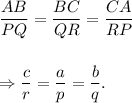
This gives

`(b)/(q)=(a)/(p).`

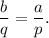
Thus, option (3) b by q is equal to a by p is correct.

Answer 2

b by q is equal to a by p

Explanation:

we know that

If triangle ABC is similar to triangle PQR

then

the ratio of their corresponding sides are equal

so

`(CB)/(RQ)=(AC)/(RP)=(AB)/(PQ)`

substitute the values

`(a)/(p)=(b)/(q)=(c)/(r)`

therefore

the answer is

b by q is equal to a by p