Answer:
 must be positive to have equal range between these functions.
must be positive to have equal range between these functions.
Explanation:
The given functions are
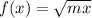 and
and

If we analyse each function, we'll notice that the range of
 is all real numbers greater of equal than zero, because a square root can't give negative values.
is all real numbers greater of equal than zero, because a square root can't give negative values.
The second funcion as the same range, all number greater or equal than zero, because it can't give a negative numbers, so they are ranges are the same.
However, their domains are

At this points, you may not notice the characteristic of
 , notice that the range of
, notice that the range of
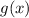 has to have a restriction for
has to have a restriction for
 , it must be greater or equal than zero, otherwise the ranges won't be the same.
, it must be greater or equal than zero, otherwise the ranges won't be the same.
Therfore,
 must be positive to have equal range between these functions.
must be positive to have equal range between these functions.