Question

Based on the image below, what is the displacement of the sound wave for Sound A + B at 0.1025 seconds?

A. -0.3316

B.0.3316

C.0.6255

D.1.6162

Answer 1

The displacement of the sound wave for Sound A + B at 0.1025 is;

D. 1.6162

Step-by-step explanation:

The sum of the displacement of two sound waves is given by the principle of superposition as follows;

y(x, t) =
`y_m`·sin(k·x - ω·t) +
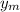`y_m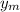`·sin(k·x - ω·t + ∅)

Therefore, by the property of the superposition of waves, the amplitudes of linear waves are added linearly when they meet at the same point at a the same time

The given parameters for the wave are;

The time at which the value of the wave amplitude are given, t = 0.1025 seconds

The displacement of the sound wave A at the given time, "t" is A = 0.9739

The displacement of the sound wave B at the given time "t" is B = 0.6423

Therefore, the displacement of the sound A + B = 0.9739 + 0.6423 = 1.6162