Question

The owner of a bakery wants to know whether customers have any preference among 5 different flavors of muffin. A sample of 200 customers provided the data below. Use a = 0.01. The table below shows how many people were tested at each location and their stated preferences Flavor Orange Blueberry Chocolate Vanilla Vanilla and Raisins # of customers 30 52 38 36 44 Calculate the chi-square test statistic X 2. Test the claim that the probabilities show no preference.

Answer 1

So,, there are 5 different flavors. A total of 180 people were asked. Hence, the hypothesis that there is no significant difference is that every flavor gets 180/5=36 flavors. x^2=
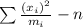
`\sum{((x_i)^2)/(m_i)}-n`. In this case, mi is the proportion of the hypothesis, thus 36, n=180 and xi is the number of actual observations. Substituting the known quantities, we get that x^2=9. The degree of association is given by
`√(X^2)/ √(N(n-1))`. This yields around 0.10, much higher than our limit.