ANSWER
The volume of the given sphere is
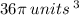
Step-by-step explanation
The volume of a sphere is calculated using the formula,
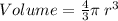
Where

is the radius of the sphere.
We substitute this value into the formula to obtain,
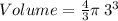
This simplifies to,
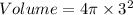
This will give us,
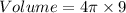
We finally multiply out to obtain,
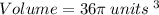
The correct answer is therefore B.