Question

You and a friend both work two different jobs. The system of linear equations represents the total earnings for xx hours worked at the first job and yy hours worked at the second job. Your friend earns twice as much as you.

One week, both of you work 4 hours at the first job. How many hours do you and your friend work at the second job?

You and your friend work _____ hour(s) at the second job.

( I may have accidentally submitted this already)

Answer 1

6

Explanation:

Answer 2

Hello, you forgot to provide the systems of equation but upon searching for the origin of this problem, I found the two equations representing the situation:

`4x+8y=64`

`8x+16y=128`

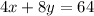
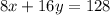
In these two equations x represents the number of hours worked at the first job and y represents the hours worked at the second job. Since you worked 4 hours for the first job, you will just substitute 4 as the value of x. You can then solve for y to find the number of hours you worked at the second job.


`4(4)+8y=64`

`16+8y=64`

`8y=48`

`y=6`

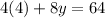
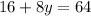
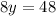
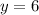
The same value will actually result by plugging 4 for x in the second equation.

ANSWER: You and your friend worked for 6 hours at the second job.