Question

does y=432(1.54) represent growth or decay? and what is the percent of increase or decrease? I need help understanding what I need to do?

Answer 1

Yes it does represent growth

Answer 2

`\bf \qquad \textit{Amount for Exponential Growth}\\\\ A=I(1 + r)^t\qquad \begin{cases} A=\textit{accumulated amount}\\ I=\textit{initial amount}\\ r=rate\to r\%\to (r)/(100)\\ t=\textit{elapsed time}\\ \end{cases}\\\\ -------------------------------\\\\ y=432(1.54)^t\implies \stackrel{A}{y}=\stackrel{I}{432}(1~+~\stackrel{r}{0.54})^t \\\\\\ r=0.54\implies r\%=0.54\cdot 100\implies r=\stackrel{\%}{54}`

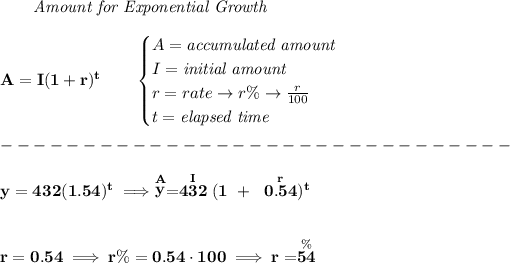
anyhow, the flag is that 1.54 is "more" than 1, thus is growth.

if it were decay, then the rate gets subtracted, and it be (1 - r), and the flag for a decay is that the value in the parentheses is less than 1, like say 432(0.95)ᵗ, since 0.95 is really just 1 - 0.05, then r = 0.05 or 0.05 * 100 or 5%.