Greetings!Find the
Solution/Point of Intersection:
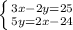 Rearrange
Rearrange the System:
 Multiply
Multiply the
First Equation by 2 and the Second Equation by 3:

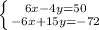 Eliminate
Eliminate the x variable:
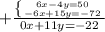
 Divide
Divide both sides by 11:
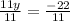
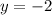 Input
Input this value into the First Equation:
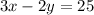
 Simplify
Simplify:
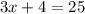
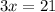 Divide
Divide both sides by 3:
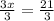
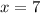 The Solution/Point of Intersection is:
The Solution/Point of Intersection is:
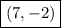
I hope this helped!
-Benjamin