Question

A falling object travels a distance given by the formula d=3t+5t2, where d is measured in feet and t is measured in seconds. How many seconds will it take for the object to travel 84 feet?

Answer 1

3.81 s

Explanation:

Let's consider the following formula that represents the journey of a falling object.

d = 3 t + 5 t²

where,

• d is the distance measured in feet

• t is the time measured in seconds

We can find the time that it will take to travel 84 feet by replacing d = 84 in the equation.

84 = 3 t + 5 t²

5 t² + 3 t - 84 = 0

We have a = 5, b = 3 and c = -84. We apply the second grade solving formula.

`x_(1,2) = \frac{-b \pm \sqrt{b^(2)-4ac} }{2a} = \frac{-3 \pm \sqrt{3^(2)-4(5)(-84)} }{2(3)}`

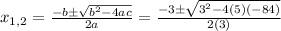
x₁ = 3.81 and x₂ = -4.41

Since the time cannot be negative, the answer is t = 3.81 s