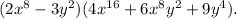Answer: The required factored form of the given expression is
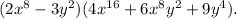
Step-by-step explanation: We are given to find the factored form of the following expression :
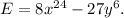
We will be using the following factorization formula :

So, the factorization of the given expression is as follows :
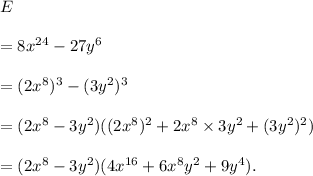
Thus, the required factored form of the given expression is