Question

Which pair of equations below represent parallel lines? and y = 9 and x = 18 y = 4x + 9 and y = 4x – 9 y = 5x + 15 and y = –5x + 15

Answer 1

Option B.

Explanation:

If two lines are in the form of y = mx + c and y' = m'x' + c'

and the given lines are parallel then their slopes will be same.

For this condition m = m'

Now we will analyse each option for the condition that the given lines are parallel lines.

Option A. y = 9 and x = 18

These lines are horizontal and vertical lines so they are not parallel.

Option B. y = 4x + 9 and y = 4x - 9

y = 4x + 9 slope of the line is m = 4

y = 4x - 9 slope of this line is m' = 4

Slopes are same so both the lines are parallel.

Option C. y = 5x + 15 and y = -5x + 15

for y = 5x + 15 slope m = 5

for y = -5x + 15 slope m' = -5

Slopes are not equal so the lines are not parallel.

Option B. is the answer.

Answer 2

The correct option is 2.

Explanation:

The slope of two parallel lines are equal.

The slope intercept form of a line is

`y=mx+b`

Where, m is slope and b is y-intercept.

In option 1, the equation of lines are

`y=9`

`x=18`

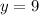
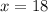
The first line is a horizontal line and the second line is a vertical line. Therefore they are not parallel line. Option 1 is incorrect.

In option 2, the equation of lines are

`y=4x+9`

`y=4x-9`

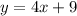
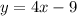
By using the slope intercept form of a line, the slope of first line is 4 and the slope of second line is 4.

Since the slope of both lines are same, therefore both lines are parallel. Option 2 is correct.

In option 2, the equation of lines are

`y=5x+15`

`y=-5x+15`

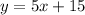
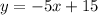
By using the slope intercept form of a line, the slope of first line is 5 and the slope of second line is -5.

Since the slope of both lines are not same, therefore both lines are not parallel. Option 3 is incorrect.