Question

What is the equation of the quadratic graph with a focus of (1 ,3) and a directrix of y=1?

Hint: y=a (x*h)^2 +k, a=1/4p (x-x1)^2 + (y-y2)^2

Answer 1

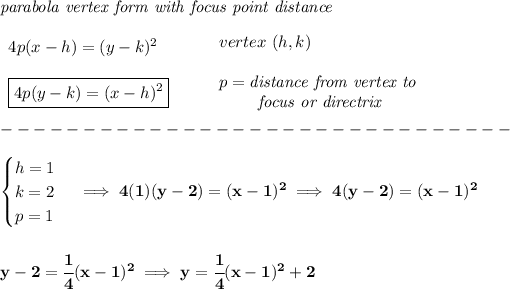
check the picture below.

now, we know the directrix is at y = 1, and the focus point is at 1,3, well, notice the picture, the distance between those fellows is just 2 units.

the vertex is half-way between those fellows, therefore, the vertex will be at 1,2.

the distance "p", from the vertex to either the directrix or focus, is really just 1 unit. Since the focus point is above the directrix, is a vertical parabola, and it opens upwards, like in the picture, and since it opens up, the "p" value is positive, or +1.


`\bf \textit{parabola vertex form with focus point distance}\\\\ \begin{array}{llll} 4p(x- h)=(y- k)^2 \\\\ \boxed{4p(y- k)=(x- h)^2} \end{array} \qquad \begin{array}{llll} vertex\ ( h, k)\\\\ p=\textit{distance from vertex to }\\ \qquad \textit{ focus or directrix} \end{array}\\\\ -------------------------------\\\\ \begin{cases} h=1\\ k=2\\ p=1 \end{cases}\implies 4(1)(y-2)=(x-1)^2\implies 4(y-2)=(x-1)^2 \\\\\\ y-2=\cfrac{1}{4}(x-1)^2\implies y=\cfrac{1}{4}(x-1)^2+2`