ANSWER
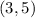
Step-by-step explanation
The given systems of equations are;
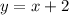
and
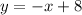
To graph the first function, which is
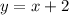
We need at least two points.
Let us use the intercepts.
At y-intercepts, x=0,
This implies that,
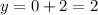
The y-intercept is,
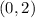
At x-intercept, y=0.
This implies that,
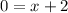

The x-intercept is
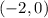
We plot the intercepts and draw a straight line through them to obtain the blue line on the graph in the attachment.
We repeat the same process for
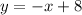
to obtain the y-intercept to be,
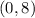
and the x-intercept to be,

We plot this one also on the the same graph to obtain the straight line in red.
The intersection of the two straight lines is
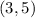
This the solution to the system of equations.