Question

If we divide x^4+4x^3+2x^2+x+4 by x^2+3x, what will be the remainder?

Answer 1

If you're familiar with synthetic division, but not the extended form (which allows you easily compute the quotient/remainder when dividing a polynomial by another polynomial of degree greater than 1), then you can perform two steps of SD.

Instead of dividing by
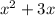
`x^2+3x`, first divide by
`x`, then by
`x+3` (since
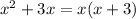
`x^2+3x=x(x+3)`). So we have

0 | 1 4 2 1 4
... | 0 0 0 0
= = = = = = = = = = = =
... | 1 4 2 1 4

which translates to


`\frac{x^4+4x^3+2x^2+x+4}x=x^3+4x^2+2x+1+\frac4x`

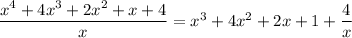
Ignoring the remainder term for now, the next round of SD yields

-3 | 1 4 2 1
... | -3 -3 3
= = = = = = = = = =
... | 1 1 -1 4

which translates to


`(x^3+4x^2+2x+1)/(x+3)=x^2+x-1+\frac4{x+3}`

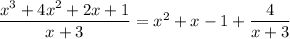
Now, putting everything together, we have


`(x^4+4x^3+2x^2+x+4)/(x^2+3x)=(x^3+4x^2+2x+1+\frac4x)/(x+3)`

`=x^2+x-1+\frac4{x+3}+\frac4{x(x+3)}`

`=x^2+x-1+(4x+4)/(x^2+3x)`

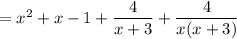
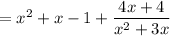
which is to say the remainder upon dividing
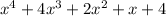
`x^4+4x^3+2x^2+x+4` by
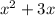
`x^2+3x` is
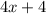
`4x+4`.