Answer:
The Perimeter is 38.25 units.
Explanation:
The vertices of trapezoid are Q(8, 8), R(14, 16), S(20, 16), and T(22, 8). we have to find the perimeter of trapezoid.
Perimeter is sum of all sides of trapezoid i.e
Perimeter=QR+RS+ST+TQ
By distance formula,
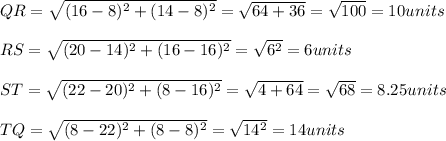
Perimeter=QR+RS+ST+TQ
=10+6+8.25+14=38.25 units.