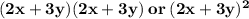Question

Factor 4x2 + 12xy + 9y2 completely.

Answer 1

Factors of the term
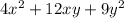`4x^2 + 12xy + 9y^2` are
`\mathbf{(2x+3y)(2x+3y)\:or\:(2x+3y)^2}`

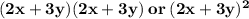
Explanation:

We need to factor the term
`4x^2 + 12xy + 9y^2`

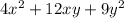
We can factor the term by breaking the middle term.

Middle term 12xy should be broken is such a way that:

• Their sum result in middle term, 12xy
• Their product result in product of first and last term i.e 4x^2(9y^2) = 36x^2y^2

Now, if we break 12xy into 6xy and 6xy

• sum of 6xy and 6xy is 12xy
• product of 6xy and 6xy is 36x^2y^2

So, our both conditions are fulfilled.

Breaking the middle terms and finding factors

`4x^2+12xy+9y^2\\=4x^2+6xy+6xy+9y^2\\=2x(2x+3y)+3y(2x+3y)\\=(2x+3y)(2x+3y)\\=(2x+3y)^2`

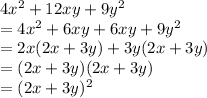
So, factors of the term
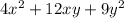`4x^2 + 12xy + 9y^2` are
`\mathbf{(2x+3y)(2x+3y)\:or\:(2x+3y)^2}`