Question

Solve for the missing side to the nearest tenth.
19
X
51

Answer 1

x = 30.2 units

Explanation:

Trigonometric Ratios

The ratios of the sides of a right triangle are called trigonometric ratios.

Selecting any of the acute angles, it has an adjacent side and an opposite side. The trigonometric ratios are defined upon those sides and the hypotenuse.

The given right triangle has an angle of measure 51° and its adjacent leg has a measure of 19 units. It's required to calculate the hypotenuse of the triangle.

We use the cosine ratio to calculate x:

`\displaystyle \cos\theta=\frac{\text{adjacent leg}}{\text{hypotenuse}}`

`\displaystyle \cos 51^\circ=(19)/(x)`

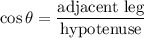
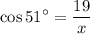
Solving for x:

`\displaystyle x=(19)/(\cos 51^\circ)`

`\displaystyle x=(19)/(0.6293)`

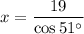
x = 30.2 units