Answer:
The point-slope form of an equation of the line is:

Explanation:
Given
Slope m = 5
Point (-2, 9)
Point slope form of an equation of a line:
Point slope form of an equation of a line is defined as

where
- m is the slope of the line
In our case,
substituting the values m = 5 and the point (x₁, y₁) = (-2, 9) in the point-slope form of the line equation

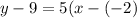

Therefore, the point-slope form of an equation of the line is:
