Question

Question is in the picture

Answer 1

(a) D = 72.05 dB (2 d.p.)

(b) I = 2.51 W/m² (2 d.p.)

Explanation:

Given function:

`\boxed{D(I)=10 \log_(10)(I)+120, \quad I\geq 0}`

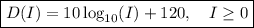
where:

• D is the sound intensity level (in dB).
• I is the sound intensity (in W/m²).

Part (a)

Given:

• 
  `I=1.6 * 10^(-5)\; \sf W/m^2`

Substitute the given value of I into the given function and solve for D:

`\begin{aligned}\implies D(1.6 * 10^(-5)) & =10 \log_(10)(1.6 * 10^(-5))+120\\& = 10 \log_(10)(0.000016)+120\\& = 10 (-4.795880017)+120\\& = -47.95880017+120\\&=72.04119983\\&=72.04\; \sf dB\;(2\:d.p.)\end{aligned}`

Part (b)

Given:

• D = 124 dB

Substitute the given value of D into the given function and solve for I:

`\begin{aligned}\implies 124 & =10 \log_(10)(I)+120\\4 & =10 \log_(10)(I)\\10 \log_(10)(I)&=4\\\log_(10)(I)&=0.4\\10^{\log_(10)(I)}&=10^{0.4\\I&=2.511886432\\I&=2.51\; \sf W/m^2\; (2\:d.p.)\end{aligned}`