Question

Multiply the polynomials (x+4)(x^2-5x+3)

Answer 1

(4x2 – 4x – 7)(x + 3)
= (4x2 – 4x – 7)(x) + (4x2 – 4x – 7)(3)
= 4x2(x) – 4x(x) – 7(x) + 4x2(3) – 4x(3) – 7(3)
= 4x3 – 4x2 – 7x + 12x2 – 12x – 21
= 4x3 – 4x2 + 12x2 – 7x – 12x – 21
= 4x3 + 8x2 – 19x – 21

Answer 2

`\\ x^(3) - x^(2) - 17x + 12`

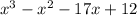
Explanation:

Write the polynomials one above the other to multiply them:

`\\ x^(2) -5x + 3\\`

`\\ x + 4\\`

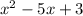
First Step

Multiply each element of
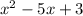`\\ x^(2) -5x + 3\\` by 4:

The result is:
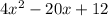`\\ 4x^(2) - 20x + 12` [1]

As it can be seen, only the coefficients are multiplied by 4, and when the operation involves multiplication with coefficients with different operators (+ or -), the following rules are crucial:

`\\ + * + = +`

`\\ - * - = +`

`\\ + * - = -`

`\\ - * + = -`

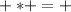
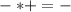
That is why 4 times -5 = -20, 4 times 1 = 4, and so 4 times 3 = 12.

Second Step

Multiply each element of
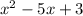`\\ x^(2) -5x + 3\\` by x:

The same rules apply here, but including the addition of powers or exponents.

The result is:
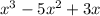`\\ x^(3) - 5x^(2) + 3x` [2].

`\\ x * x^(2) = x^(3)` or
`\\ x * x^(2) = x^(1+2) =x^(3)`

`\\ x * (-5x)=-5x^(2)`

`\\ x*3 = 3x`

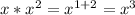
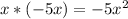
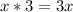
Third Step

Sum all similar terms of the previous results [1] and [2].

The result is:
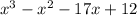`\\ x^(3)-x^(2)-17x +12`, because

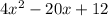
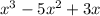
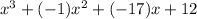
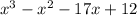`\\ 4x^(2) - 20x + 12`

`\\ x^(3) - 5x^(2) + 3x`

__________________________

`\\ x^(3) + (4-5)x^(2) + (-20+3)x+12`

`\\ x^(3) + (-1)x^(2) + (-17)x+12`

`\\ x^(3) - x^(2) -17x+12`.