Answer: b. 5
Explanation:
Given : The function
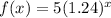 ,models the length (cm) of a lizard from the beginning of its life.
,models the length (cm) of a lizard from the beginning of its life.
Here the length of the lizard depends upon the time from the beginning, so x must be representing the time period from the beginning .
The general exponential equation is given by :-
 , where A is the initial value , b is the growth factor and x is the time.
, where A is the initial value , b is the growth factor and x is the time.
Comparing the given equation to the general equation , we get, the length of the lizard at the beginning of its life = 5 cm
Hence, 5 represents the length of the lizard at the beginning of its life .