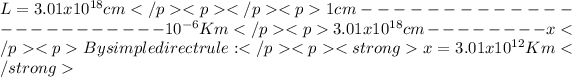Question

A typical virus is 5x10-6 cm in diameter. if avogadro's number of these virus particles were laid in a row, how many kilometers long would the line be?

Answer 1

`3.01x10^(12) Km`

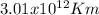
Step-by-step explanation:

The avogrado's number is
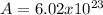`A = 6.02x10^(23)`, which means that in one mol of a substance, there is this number of molecules, or atoms, for example.

So, to know how long would be the line (L), we must multiply the numbers of diameter and avogrado:

`L = 5x10^(-6) x 6.02x10^(23)`

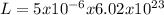
To do it, we must multiply the numbers, repeat the basis 10 and add the exponents, then:

`L = 3.01x10^(18) cm </p><p></p><p>1 cm ------ ------------------10^(-6) Km</p><p>3.01 x 10^(18) cm --------x</p><p>By simple direct rule: </p><p><strong>x = 3.01x10^(12) Km</strong>`

Answer 2

Answer is: line be long 3,011·10¹³ kilometers.
diametar of virus = 5·10⁻⁶ cm ÷ 100000 = 5·10⁻¹¹ km.
line lenght = 5·10⁻¹¹ km · 6,023·10²³.
line lenght = 3,011·10¹³ km.
Avogadro number = 6,023·10²³.
1 cm = 10⁻² m = 10⁻⁵ km.