Question

Skylar plans to use $3400 to open a savings account with an annual interest rate of 1.15%. How much more interest will he earn over 13 years if he chooses a compound interest account that compounds interest quarterly instead annually? Round your answer to the nearest cent.

interest compounded annually: A = P (1 + r)t
interest compounded quarterly: A = P (1 +r/4)4t

Answer 1

$ 2.5

Explanation:

Given that

Total Amount to be invested = P = $3400

Total Time of investment = t = 13 years

Rate of interest = r = 1.15 %

Lets calculate Simple interest first

As we know formula for simple interest is

`A = P (1 +r)^(t)`

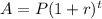
Substituting the values

`A = 3400(1+ (1.15)/(100) )^(13)`

A = $ 3944.9

Lets now calculate the compound interest

As we know formula for simple interest is

`A = P (1 + (r)/(n))^(nt)`

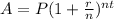
As compound interest applies every 3 months so n =3

So,

`A = 3400 (1 + (1.15)/(100*4) )^(13*4)`

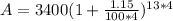
A = $ 3947.4

Now Lets calculate how much more he will earn using compound interest.

It can be found by taking difference of compound interest and simple interest

Amount = 3947.4 - 3944.9 = $ 2.5

So Skyler must have earned 2.5 dollar more using the compound interest.