Question

If two angles form a linear pair and then m<1=2x+20 and m<2=48x find the measure of each angle

Answer 1

Angle 1= 26.4
Angle 2= 153.6

Answer 2

Linear pair states that it is the pair of adjacent angle formed when two lines intersect.

If two angles form a linear pair then their sum add up to 180 degree.

Given that:

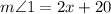`m\angle 1 = 2x+20` and
`m\angle 2 = 48x` are two angles form a linear pair.

Then by definition of linear pair;

`m\angle 1+m\angle 2 = 180^(\circ)`

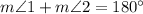
Substitute the given values and solve for x;

`2x+20+48x = 180^(\circ)`

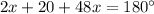
Combine like terms;

`50x+20= 180^(\circ)`

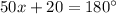
Subtract 20 from both sides we get;

`50x= 160^(\circ)`

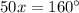
Divide both sides by 50 we get;

`x = 3.2`

Then; the measure of these angles are;

`m\angle 1 = 2(3.2)+20 = 6.4+20 =26.4^(\circ)`

`m\angle 2 = 48(3.2)= 153.6^(\circ)`