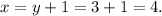Answer:
4 brothers and 3 sisters
Explanation:
Let x be the number of brothers and y be the number of sisters in the family.
1. Each brother has x-1 brothers and y sisters. If each brother has as many sisters as brothers, then
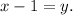
2. Each sister has y-1 sisters and x brothers. If each sister has twice as many brothers as she has sisters, then
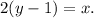
3. Solve the system of two equations:

Then